Quantum Numbers II
What does the third quantum number mean?
The number m also has a connection with angular momentum, but it's not
necessary to know the details of that in order to make some sense of
m's significance. The key point about m is that it does not
affect the electron's energy, although, as you've seen, it does change the
shape of the electron cloud.
So when Bob said before that there could be
different kinds of clouds at the same energy, what he meant was that there
could be different values of m for the same n and l.
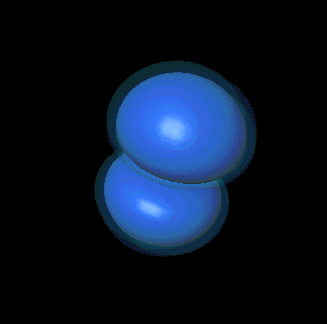
That's absolutely right. For example, here are the quantum numbers for the
two different p states Bob showed you:
|
|
|
|
|
That reminds me of some other questions I had. First, Bob said that the number of sublevels keeps
going up with each primary level. Can you explain that, in terms of quantum
numbers?
What's happening is that there are restrictions on the possible values of each
quantum number. n is allowed to be any positive integer. Within the
level given by a particular n, l can take on only integer values
from 0 to n-1.
So when n is 1, l can only be 0, and that's why the
first row has only s states. Then when n=2, l can
be either 0 or 1, and that gives you s and p--I get it!
I also remember Bob saying that there's
only one kind of s state, three kinds of p states, five kinds of d states, and
so on. Does that mean there are also restrictions on what m can be?
Very good. Given a particular l, m is entitled to be any
integer from minus l up to l. For example, when l=1,
m can be -1, 0, or 1; those are your three p states. If you work it
out, you'll see that for a given l, there are 2l+1 different
values of m.
